
SEQUENCE FORMULA SERIES
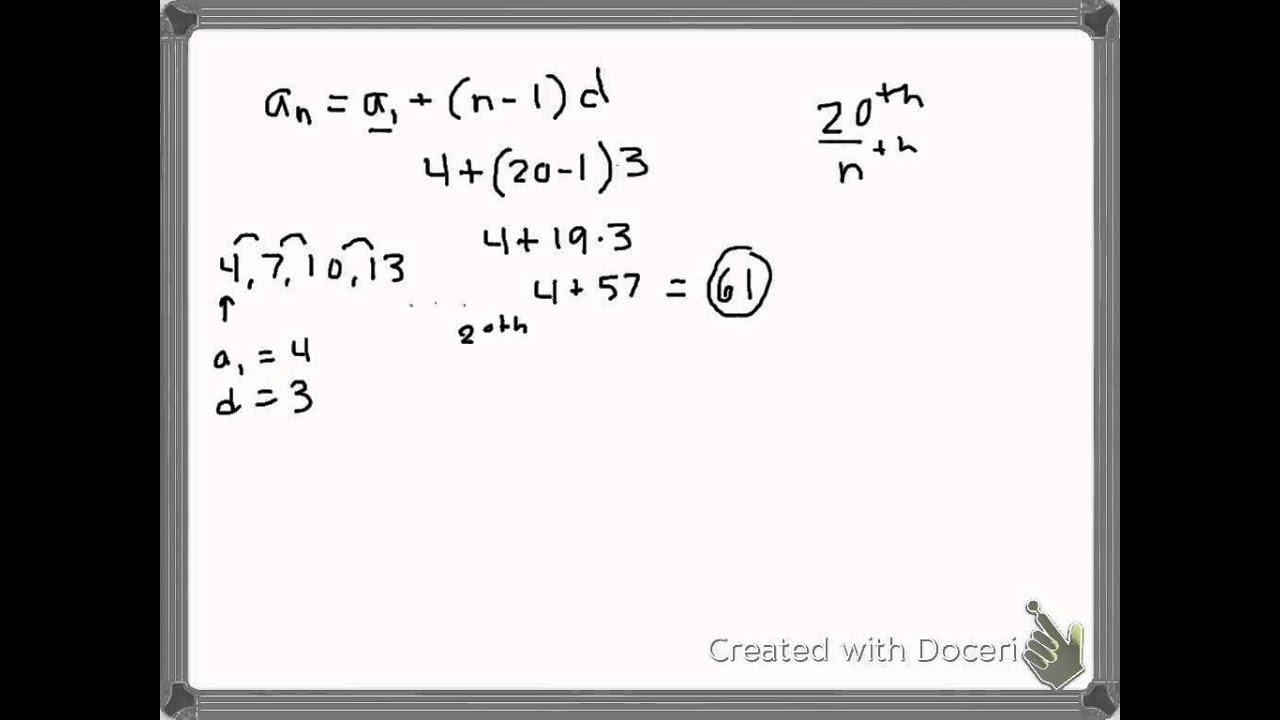
To make work much easier, sequence formula can be used to find out the last number (Of finite sequence with the last digit) of the series or any term of a series. That is each subsequent number is increasing by 3. To recall, all sequences are an ordered list of numbers. \(1,\ 2,\ 3,\ 4,\dots\) signifies the position of the term in the sequence. Sequence formula mainly refers to either geometric sequence formula or arithmetic sequence formula.
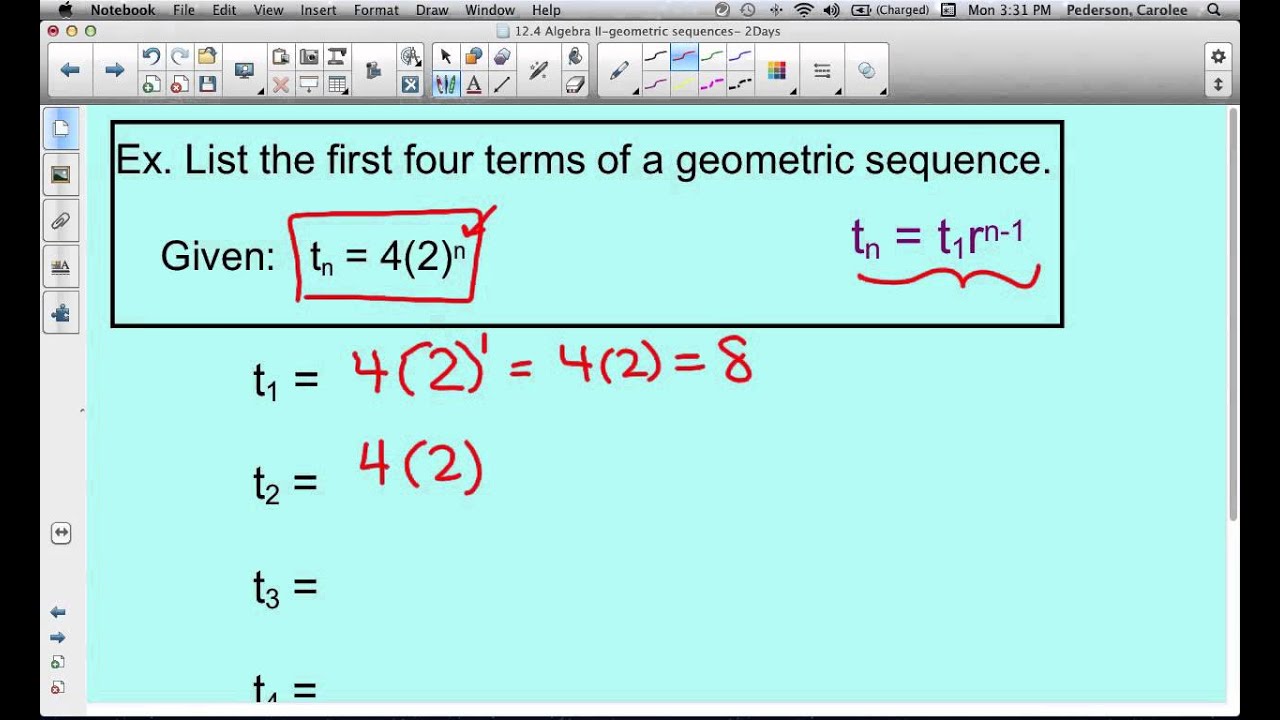
SequenceĪ sequence is an organisation of any objects/elements/set of digits in a particular order accompanied by some rule.įor example, if \(a_1,\ a_2,\ a_3,\ a_4,\dots\dots\dots\) etc indicate the terms of a sequence, then. A sequence is also recognised as progression on the other hand, a series is generated by the sequence. Sequence and series are employed in basic to higher-level mathematical concepts. If you are reading Sequence and Series then also go through the Number System. What Is a Recursive Formula? The recursive formula is defined by how the previous term, $a_ĭouble-check the validity of the recursive formula by checking if it still applies for the next few terms of the sequence.Sequence and series contribute a major part of mathematics, where the arrangement of objects or items in a progressive manner is termed as a sequence and the sum of all the terms in the particular sequence is termed as a series(possessing a definite relationship among all the terms/objects of the sequence). This means is the first term, and is the term before the term. Write the formula for the nth term of the sequence and use it to find the number of logs in the 76th row. In the formula, is any term number and is the term. Identify the pattern in the sequence of numbers. By the end of this article, we want you to feel confident when working on different problems involving recursive formulas! Here is a recursive formula of the sequence along with the interpretation for each part. In our discussion, we will be showing how arithmetic, geometric, Fibonacci, and other sequences are modeled as recursive formulas.
SEQUENCE FORMULA HOW TO
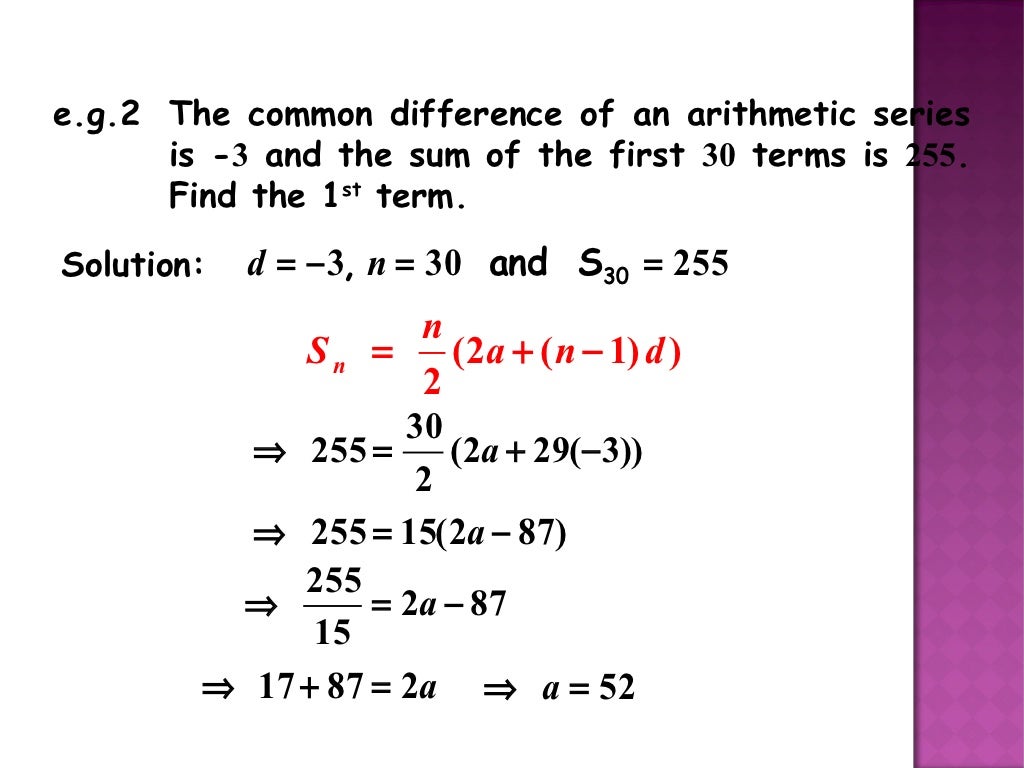
This is also why knowing how to rewrite known sequences and functions as recursive formulas are important. The recursive formula has a wide range of applications in statistics, biology, programming, finance, and more. In addition, we will explore several examples with answers to understand the application of the arithmetic sequence formula. Positive and negative SEQUENCE can work with both positive and negative values. The result is 50 numbers starting at 0 and ending at 147, as shown in the screen. Here, we will look at a summary of arithmetic sequences. SEQUENCE(10,5,0,3) With this configuration, SEQUENCE returns an array of sequential numbers, 10 rows by 5 columns, starting at zero and incremented by 3. This formula allows us to find any number in the sequence if we know the common difference, the first term, and the position of the number that we want to find. We define the recursive formula based on how the previous term affects the next term. Arithmetic sequences exercises can be solved using the arithmetic sequence formula. We can observe recursive formulas and recursion in our daily lives – this includes recording our savings and expenses, monitoring our progress in school, and even observing the number of sunflower petals! Learning about recursive formulas allows us to work with functions and sequences that are defined by observing the behavior between two succeeding terms. This lesson will work with arithmetic sequences, their recursive and explicit formulas and finding terms in a sequence.


 0 kommentar(er)
0 kommentar(er)
